Imagine beams of light coming from an emitter and travelling around a circular path in both directions, until they arrive back at the source. Such an arrangement can be constructed by using an optic fibre in a circular loop, injecting light at both ends. The distance travelled by the clockwise beam is the same as the distance travelled by the anticlockwise beam, and the speed of light in both directions is the same, so the time taken for each beam to travel from the source back to the origin is the same. So far, so good.
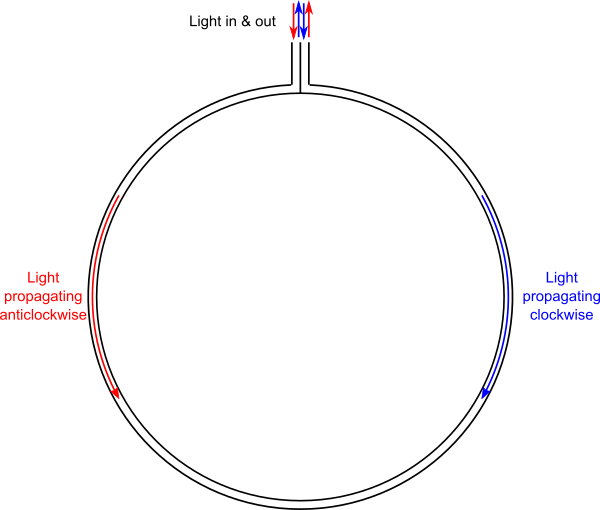
Now imagine the whole thing is rotating – let’s say clockwise. For reference we’ll use the numbers on a clock face and the finer divisions into 60 minutes. The optic fibre ring runs around the edge of the clock, with the light source and a detector at 12. Now imagine that the clock rotates fast enough that by the time the clockwise-going light reaches the original 12 position, the clock has rotated so that 12 is now located at the original 1 minute past 12 position. The light has to travel an extra 60th of the circle to reach its starting position (actually a tiny bit more than that because the clock is still rotating and will have gone a tiny bit further by the time the light beam catches up). But the light going anticlockwise reaches the source early, only needing to travel a tiny bit more than 59/60 of the circle. The travel times of the two beams of light around the circle are different.
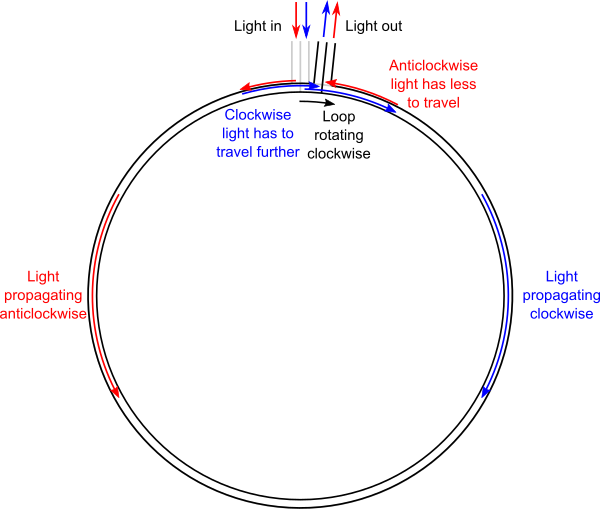
This is a very simplified explanation, and figuring out the mathematics of exactly what happens involves using special relativity, since the speed of light is involved, but it can be shown that there is indeed a time difference between the travel times of beams of light heading in opposite directions around a rotating loop. The time difference is proportional to the speed of rotation and to the area of the loop (and to the cosine of the angle between the rotation axis and the perpendicular to the loop, for those who enjoy vector mathematics). This effect is known as the Sagnac effect, named after French physicist Georges Sagnac, who first demonstrated it in 1913.
Measuring the minuscule time difference between the propagation of the light beams is not difficult, due to the wave nature of light itself. The wavelength of visible light is just a few hundred nanometres, so even a time difference of the order of 10-16 seconds can be observed because it moves the wave crests and troughs of the two beams relative to one another, causing visible interference patterns as they shift out of synchronisation. This makes the device an interferometer that is very sensitive to rotational speed.
The Sagnac effect can be seen not only in a circular loop of optic fibre, but also with any closed loop of light beams of any shape, such as can be constructed with a set of mirrors. This was how experimenters demonstrated the effect before the invention of optic fibres. Because the paths of the two beams of light are the same, just reversed, a Sagnac interferometer is completely insensitive to mechanical construction tolerances, and only sensitive to the physical rotation of the device.
Sagnac actually performed the experiment in an attempt to prove the existence of the luminiferous aether, a hypothetical medium permeating all space through which light waves propagate. He believed his results showed that such an aether existed, but Max von Laue and Albert Einstein showed that Sagnac’s effect could be explained by special relativity, without requiring any aether medium for light propagation.
The interesting thing about the Sagnac effect is that it measures absolute rotational speed, that is: rotation relative to an inertial reference frame, in the language of special relativity. In practice, this means rotation relative to the “fixed” position of distant stars. This is useful for inertial guidance systems, such as those found on satellites, modern airliners and military planes, and missiles. The Sagnac effect is used in ring laser gyroscopes and fibre optic gyroscopes to provide an accurate measure of rotational speed in these guidance systems. GPS satellites use these devices to ensure their signals are correctly calibrated for rotation – without them GPS would be less accurate.
Because the magnitude of the Sagnac effect depends on both the rotational speed and the area of the light loop, by making the area large you can make the interferometer incredibly sensitive to even very slow rotation. Rotations as slow as once per 24 hours. You can use these devices to measure the rotation of the Earth.
This was first done in 1925. Albert A. Michelson (of the famous Michelson-Morley experiment that disproved the existence of the luminiferous aether), Henry G. Gale, and Fred Pearson acquired the use of a tract of land in Clearing, Illinois (near Chicago’s Midway Airport), and built a huge Sagnac interferometer, a rectangle 610×340 metres in size [1][2].
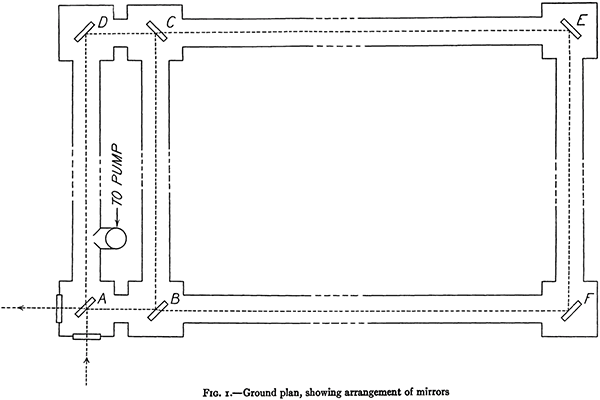
With this enormous area, the shift in the light beams caused by the rotation speed of the Earth at the latitude of Chicago was around one fifth of a wavelength of the light used – easily observable. The Michelson-Gale-Pearson experiment’s measurements and calculations showed that the rotation speed they measured was consistent with the rotation of the Earth once every 23 hours and 56 minutes – a sidereal day (i.e. Earth’s rotation period relative to the stars; this is shorter than the average of 24 hours rotation relative to the sun, because the Earth also moves around the sun).
Now the interesting thing is that the Sagnac effect measures the linear rotation speed, not the angular rotation rate. The Earth rotates once per day – that angular rotation rate is constant for the entire planet, and can be modelled in a flat Earth model simply by assuming the Earth is a spinning disc, like a vinyl record or Blu-ray disc. But the linear rotation speed of points on the surface of the Earth varies.
In the typical flat Earth model with the North Pole at the centre of the disc, the rotation speed is zero at the North Pole, and increases linearly with distance from the Pole. As you cross the equatorial regions, the rotational speed just keeps increasing linearly, until it is maximal in regions near the “South Pole” (wherever that may be).

On a spherical Earth, in contrast, the rotation speed is zero at the North Pole, and varies as the cosine of the latitude as you travel south, until it is a maximum at the equator, then drops again to zero at the South Pole.
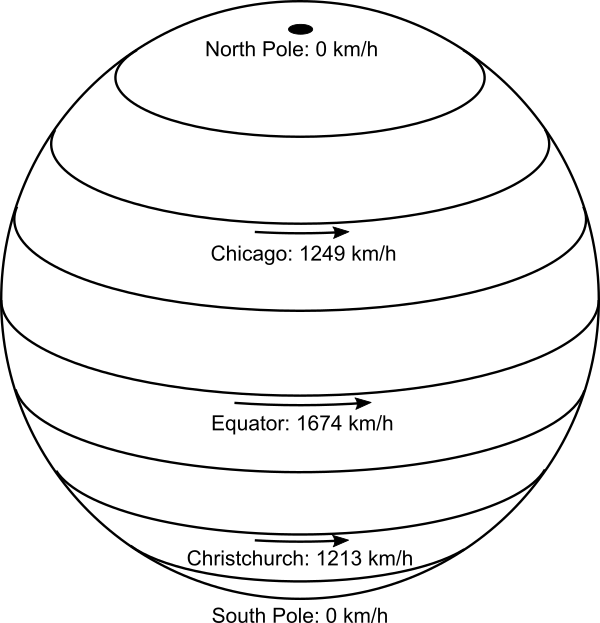
Here is a table of rotation speeds for the two models:
| Latitude | Speed (km/h) Flat model |
Speed (km/h) Spherical model |
|---|---|---|
| 90°N (North Pole) | 0.0 | 0.0 |
| 60°N | 875.3 | 837.2 |
| 41.77°N (Clearing, IL) | 1407.2 | 1248.9 |
| 30°N | 1750.5 | 1450.1 |
| 0° (Equator) | 2625.8 | 1674.4 |
| 30°S | 3501.1 | 1450.1 |
| 45.57°S (Christchurch) | 3896.6 | 1213.3 |
| 60°S | 4376.4 | 837.2 |
| 90°S (South Pole) | 5251.6 | 0.0 |
In the Michelson-Gale-Pearson experiment, the calculated expected interferometer shift was 0.236±0.002 of a fringe (essentially a wavelength of the light used), and the observed shift was 0.230±0.005 of a fringe. The uncertainty ranges overlap, so the measurement is consistent with the spherical Earth model that they used to calculate the expected result.
If they had used the North-Pole-centred flat Earth model, then the expected shift would have been 1407.2/1248.9 larger, or 0.266±0.002 of a fringe. This is well outside the observed measurement uncertainty range. So we can conclude that Michelson’s original 1925 experiment showed that the rotation of the Earth is inconsistent with the flat Earth model.
Nowadays we have much more than that single data point. Sagnac interferometers are routinely used to measure the rotation speed of the Earth at various geographical locations. In just one published example, a device in Christchurch, New Zealand, at a latitude of 43°34′S, measured the rotation of the Earth equal to the expected value (for a spherical Earth) to within one part in a million [3]. Given that the expected flat Earth model speed is more than 3 times the spherical Earth speed at this latitude—and all of the other rotation speed measurements made all over the Earth consistent with a spherical Earth—we can well and truly say that any rotating disc flat Earth model is ruled out by the Sagnac effect.
References:
[1] Michelson, A. A. “The Effect of the Earth’s Rotation on the Velocity of Light, I.” The Astrophysical Journal, 61, 137-139, 1925. https://doi.org/10.1086%2F142878
[2] Michelson, A. A.; Gale, Henry G. “The Effect of the Earth’s Rotation on the Velocity of Light, II.” The Astrophysical Journal, 61, p. 140-145, 1925. https://doi.org/10.1086%2F142879
[3] Anderson, R.; Bilger, H. R.; Stedman, G. E. “ “Sagnac” effect: A century of Earth‐rotated interferometers”. American Journal of Physics, 62, p. 975-985, 1994. https://doi.org/10.1119/1.17656
Loving this series, hope you do turn it into a book. It worked for Randall Munroe….
Keep it up!
Strangely, Wikipedia currently says that the Sagnac effect depends on the angular velocity, not the linear velocity. And the Michaelson et al. paper gives the phase difference between the light passing the loop in opposite directions as 4 A omega sin(phi) / lambda V, where A is the area of the loop, omega the angular velocity, phi the latitude, lambda the wavelength of the light, and V the speed of light. The radius of the Earth dropped out of their derivation. What matters is the angle between the plane of the loop and the axis of rotation. The effect is strongest at the poles, where sin(phi) is large, and zero at the equator.
This still distinguishes a flat Earth from a spherical Earth, though. In a flat Earth, the horizontal is always perpendicular to the axis of rotation, and so the effect would be constant everywhere.
It depends on how you break it down. The formula on Wikipedia uses ω sin φ as you noted, but it could just as easily have used v/r, where v is the linear velocity and r is the radius of Earth. This is because v = ωρ (where ρ is the distance from Earth’s axis of rotation), and ρ = r sin φ.
Except that in the formula (from the Michelson paper), phi is the latitude, not the angle from the pole, as is more usual in spherical coordinates. So rho = r cos phi, not r sin phi. The important factor is rate of change of linear velocity with respect to north-south displacement, not the linear velocity itself. This rate of change is greatest at the poles, and least at the equator.
H'm, you're right; ρ = r cos φ. You could still say v/r tan φ in place of ω sin φ, but that seems like a less reasonable way to put it (with a sign difference). That said, the rate of change of linear velocity with respect to north-south displacement, using v = ωr cos φ and taking ω and r as constant, is dv = −ωr sin φ dφ divided by d(rφ) = r dφ, which comes to −ω sin φ, so there are still two ways to put it. But the way involving linear velocity needs Calculus to explain, while the way involving angular velocity needs only Algebra, so I can see why Michelson et al might choose the latter. (I just skimmed their 6-page paper, cited in the Wikipedia article, and they don’t seem to give any explanation for their formula that you quoted. Although I did notice that, as late as 1925, they felt it necessary to explain that the formula was correct assuming either a stationary luminiferous aether *or* special relativity.)
[I hope that the character entities are accepted here; if this is garbled, then I will repost.]
David’s explanation of the Sagnac effect definitely shows it as relating to the angular velocity; the configuration of his ring of light pipes in relation to the rotation is the one we would observe at the poles, with the plane of the pipes being the plane of rotation. At the equator, the pipes would be rotating about an axis in the plane of the loop they form (while moving at speed in a perpendicular direction parallel to the plane of the loop). So the effect as explained would be maximal at the poles and minimal at the equator. David hasn’t at all explained how the effect is about linear velocity rather than angular velocity. I think I need to go and derive this effect for myself …
Hi all, thanks for your comments. I’m not by any means an expert on the Sagnac effect, and I may well have gotten a bit confused in my reading about it. I’ll take a close look at it again when I get time and perhaps revise this post.
OK, Michelson’s sin φ term is from the inner product of the angular velocity vector (in the direction of Earth’s spin axis) and the area vector (perpendicular to the Earth’s surface at Clearing Illinois), which is indeed the sin of the latitude (so zero at the equator and 1 at the pole) or cos of the angle from the axis. Of course, nothing requires us to arrange our loop in the local surface of the Earth; it can be upright or angled instead; what matters is the component of area as projected onto a plane perpendicular to the spin axis. So build your loop on the North- or South-facing side of a big building near the equator to get a measurable result there. All the same, the w in question is the Earth’s spin angular velocity, one turn per (sidereal) day.
For Sagnac effect detectors in our hand-held electronic pieces of wizardry (I read that the term “gyrolaser” gets used), I imagine we actually use three loops about perpendicular axes, since it would be silly to make assumptions about the orientation in which the user is holding their device ! That would give us all three components of the angular momentum vector, which is what we’d surely want in any case.
So the thing we can measure with Sagnac-effect detectors is the Earth’s spin vector (angular velocity and direction of axis); and we can show that this is parallel to Earth’s surface at the equator and climbs to upright at each pole. Presumably – I haven’t done the experiment or found reports from those who have ;^>
For a flat Earth, if it does any spinning at all (rather than being the one true fixed and invariant centre of the yadda), what would matter is the inner product of its spin vector (along the axis of spin) and normal to the plane of the loop traversed by the light; in Michelson’s case, the vertical component; and this would produce the same result at any location on the flat Earth, regardless of latitude.
Of course, for our pocket devices, the rotations caused by the user’s motion shall dwarf the Earth’s spin (descending a stair-way, I make several turns in less than a minute, rather than one per day); however, provided the device is sensitive enough and can integrate up the cumulative rotation, I guess it can keep track of how it’s oriented relative to Earth’s spin axis. (Likewise, by the way and contrary to popular myth, the Coriolis effect is *NOT* responsible for the water in your bath or toilet circulating: little disturbances due to the inflowing water not coming in perfectly symmetrically, or what you’ve done in the water disturbing it asymmetrically, shall dwarf the Coriolis term, which is of the cross product of the velocity of flow with the Earth’s spin, so would tend to take of order a day to cause a significant change in the direction of the flow. I hope your bath empties faster than that !)
One paper I dug up (here: https://arxiv.org/pdf/1110.1643.pdf as PDF) looks into the next order corrections due to relativistic effects. The base term c.Δt = 4.A·w/c gets scaled by a power-series with leading-order term 1 and later-order terms in powers of v.v/c/c (which is typically tiny); for the v in these terms, apparently (Section 3) the velocity relative to the spin axis is what matters, so (as long as we measure using a detector in a plane perpendicular to Earth’s spin axis) the higher linear speed at low latitudes would make these higher-order terms more significant. However, the paper is quite clear that these higher-order terms are, even then, only measurable with the most sensitive lab equipment we might soon build; so I doubt our pocket-gadgets are using them. For now ;^)
Another paper (here: https://arxiv.org/pdf/gr-qc/0401005.pdf as PDF) does show that the speed of the emitter/observer of the light matters, but that’s speed relative to the trajectory the light is traversing, so still won’t make any difference (to the currently-detectable leading-order term) based on where you do the experiment on a spinning globe and the loop is moving with you, aside from a slight effect due to you being at the edge of the loop, while your shared rest-frame spins (and what matters is your distance from the loop, not from the spin axis).
The effect (to leading order) is equally well explained by relativistic theory or luminiferous æther !
I note that the situation is further complicated by the orders of magnitudes of terms. Michelson’s 2010′ by 1113′ field at 41°46′ N spans 25ish seconds of arc east-west and nearly 11 seconds north-south; these lead to perturbations we can’t ignore when considering a λ.Δ (i.e. c.Δt) on the order of 17 parts in a million million (17.64e-12) of the length of the circuit the light traverses. The analysis *must* take account of differences in length between the nearer-pole and nearer-equator sides of the loop; assuming it’s a rectangle would be bogus. Further study to come !
(I confess I’m enjoying this ;^)
(hmm … well, or equivalently: if the north side and south side were actually of equal length, then we *must* take account of the other two sides not actually being north-south.)
The first of the two Michelson papers that David M-M references seems to be using a “rectangle” whose sides are segments of, alternately, two parallels of latitude, and two meridians.
Michelson and Gale measured the CORIOLIS EFFECT, which is proportional to the area of the interferometer, and not the SAGNAC EFFECT, which is proportional to the velocity (and thus to the radius of rotation).
Here is the derivation of the Coriolis effect formula featured in the 1925 paper published by A. Michelson:
https://www.ias.ac.in/article/fulltext/pram/087/05/0071
Spinning Earth and its Coriolis effect on the circuital light beams
The final formula is this:
dt = 4ωA/c^2
The SAGNAC EFFECT for the MGX or for the ring laser gyroscopes is much larger than the CORIOLIS EFFECT, since the Sagnac effect now is proportional to the radius of rotation.
According to Stokes’ rule an integration of angular velocity Ω over an area A is substituted by an integration of tangential component of translational velocity v along the closed line of length L limiting the given area.
That is, the form of the correct Sagnac effect must be: 2VL/c^2
V = angular velocity x radius of the Earth
However, for the MGX we have two velocities, one for each latitude, and two lengths for the each side of the interferometer (large sides).
Here is the correct formula for the SAGNAC EFFECT for the MGX:
dt = 2(V1L1 + V2L2)/c^2
Michelson and Gale measured ONLY the Coriolis effect and NOT the Sagnac effect which is thousands of times larger than the Coriolis formula.
I love that flat Earthers literally did this experiment, found out that the Earth was in fact spinning, and denied it anyway.
About the Sagnac effect and relativistic physics. There is a reason that at the time Sagnac thought that his experiment had vindicated the supposition of a luminiferous aether. The Sagnac effect is unique in the following way: it does not distinguish between aether-type theory and relativistic theory. That is: in terms of either framework the prediction of the outcome *is the same*. Kevin Brown offers the following consideration: the fact that you have counterpropagating light traversing the same path gives a lot of cancelation. The way I understand that: the cancelation is so extensive that even relativistic effect cancels out. (What remains is topology.) So: uniquely the Sagnac effect cannot be used as a test of relativistic physics.
As pointed out by Edward Welbourne, the Sagnac effect relates exclusively to rotation. About the Michelson-Gale-Pearson setup: the plane of that interferometry setup was – inevitably – at an angle to the plane of the equator. The amount of phase shift that is obtained is proportional to the component of rotation parallel to the plane of the Equator. Analogy: the precession of the plane of swing of a Foucault pendulum. The closer you are to the Equator the slower the rate of precession; only the projection onto the plane of the Equator contributes to the Foucault effect.
The Michelson-Gale-Pearson setup had to be so large because they had to obtain an interference effect from a phase shift.
A ring laser interferometer with capability to monitor the Earth rotation with high accuracy can be much smaller because in the case of a ring laser interferometer the interference effect that is elicited is a beat frequency.
There is a 2022 article by a group at the University of Pisa.
They have in operation a 1.6 meter by 1.6 meter ring laser interferometer. The setup is slanted to the local horizontal, to align with the equatorial plane. For that setup (Helium-Neon laser) the beat frequency that is obtained+ is 184 Hz
A high sensitivity tool for geophysical applications: A geometrically locked Ring Laser Gyroscope. https://arxiv.org/pdf/2207.04942.pdf